|
In the complex 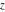 ‐plane, the function ‐plane, the function 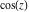 is defined using the exponential function is defined using the exponential function 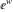 in the points in the points 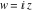 and and 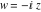 through the formula: through the formula: The key role in this definition of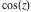 belongs to the famous Euler formula that connects the exponential, the sine, and the cosine functions: belongs to the famous Euler formula that connects the exponential, the sine, and the cosine functions: Changing 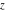 to to 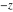 , the Euler formula can be converted into the following modification: , the Euler formula can be converted into the following modification: Adding the preceding formulas gives the following result: Here are two graphics showing the real and imaginary parts of the cosine function over the complex plane.
|