|
Introduction to the Airy functions
General
In 1838, G. B. Airy investigated the simple-looking differential equation:
This is quite similar to the differential equation 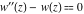 for the hyperbolic sine and hyperbolic cosine functions, which has the general solution for the hyperbolic sine and hyperbolic cosine functions, which has the general solution 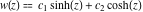 . Airy built two partial solutions . Airy built two partial solutions 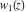 and and 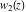 for the first equation in the form of a power series for the first equation in the form of a power series 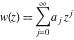 . These solutions were named the Airy functions. Much later, H. Jeffreys (1928–1942) investigated these functions more deeply. The current notations Ai and Bi were proposed by J. C. P. Miller (1946). . These solutions were named the Airy functions. Much later, H. Jeffreys (1928–1942) investigated these functions more deeply. The current notations Ai and Bi were proposed by J. C. P. Miller (1946).
The Airy functions 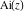 and and  are the special solutions of the differential equation: are the special solutions of the differential equation:
satisfying the following initial conditions:
These functions have different equivalent representations in the form of series or generalized hypergeometric functions. The hypergeometric representation can be conveniently used as a definition of the Airy functions.
Definition of Airy functions
The Airy functions 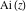 and and 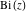 , and their derivatives , and their derivatives 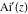 and and 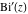 can be defined by: can be defined by:
A quick look at the Airy functions Here is a quick look at the graphics for the Airy functions and their derivatives along the real axis.
Connections within the group of Airy functions and with other function groups
Representations through more general functions
The Airy functions 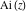 and and 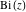 , and their derivatives , and their derivatives 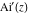 and and 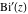 are particular cases of the more general Bessel, hypergeometric, and Meijer G functions. are particular cases of the more general Bessel, hypergeometric, and Meijer G functions.
The Airy functions can be represented as combinations of modified Bessel functions 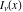 with argument with argument 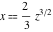 and parameter and parameter 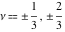 through the formulas: through the formulas:
Airy functions and their derivatives can be expressed as particular cases of single generalized Meijer G functions:
They cannot be represented as cases of single classical Meijer G functions. Such representations include combinations of two classical Meijer G functions:
The best-known properties and formulas for Airy functions
Real values for real arguments
For real values of argument 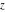 , the values of the Airy functions , the values of the Airy functions 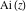 and and  , and their derivatives , and their derivatives 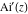 and and 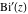 are real. are real.
Simple values at zero
The Airy functions 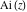 and and 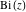 , and their derivatives , and their derivatives 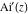 and and 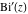 have rather simple values for argument have rather simple values for argument 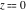 : :
Analyticity
The Airy functions 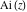 and and 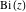 , and their derivatives , and their derivatives 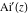 and and 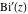 defined for all complex values of defined for all complex values of 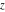 , are analytic functions of , are analytic functions of 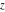 over the whole complex over the whole complex 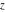 ‐plane, and do not have branch cuts or branch points. These functions are entire functions with an essential singular point at ‐plane, and do not have branch cuts or branch points. These functions are entire functions with an essential singular point at 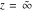 . .
Periodicity
The Airy functions  and and 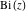 , and their derivatives , and their derivatives 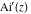 and and 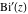 are not periodic functions. are not periodic functions.
Parity and symmetry
The Airy functions 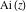 and and 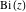 , and their derivatives , and their derivatives 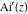 and and 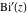 have mirror symmetry: have mirror symmetry:
Series representations
The Airy functions  and and 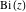 , and their derivatives , and their derivatives 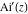 and and 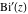 have rather simple series representations at the origin: have rather simple series representations at the origin:
These series converge at the whole 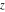 ‐plane and their symbolic forms are the following: ‐plane and their symbolic forms are the following:
Two sums in the previous formulas can be combined into one formula, and the resulting formulas can be rewritten as follows:
Interestingly, closed-form expressions for the truncated version of the Taylor series at the origin can be expressed in terms of the generalized hypergeometric function 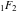 , for example: , for example:
Asymptotic series expansions
The asymptotic behavior of the Airy functions 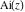 and and 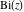 can be described through formulas that depend on the sector of can be described through formulas that depend on the sector of 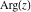 (Stokes phenomenon). The following formulas are examples of asymptotic expansions that are valid for (Stokes phenomenon). The following formulas are examples of asymptotic expansions that are valid for 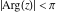 (for (for 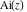 and and  ) and for the sector ) and for the sector 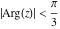 (for (for 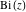 and and 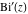 ): ):
By using discontinuous functions such as 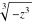 , it is possible to write single expansions that are valid for all directions: , it is possible to write single expansions that are valid for all directions:
Integral representations
The Airy functions 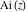 and and 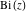 , and their derivatives , and their derivatives 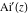 and and 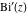 have rather simple integral representations through sine, cosine, and power functions: have rather simple integral representations through sine, cosine, and power functions:
Transformations
The argument of the Airy functions 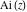 and and 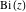 , and their derivatives , and their derivatives 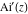 and and 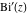 can be simplified for third roots: can be simplified for third roots:
Simple representations of derivatives
The derivatives of the Airy functions 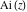 and and 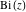 , and their derivatives , and their derivatives 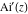 and and 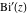 have simple representations that can also be expressed through Airy functions: have simple representations that can also be expressed through Airy functions:
The symbolic 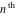 -order derivatives have more complicated representations in terms of the regularized hypergeometric function -order derivatives have more complicated representations in terms of the regularized hypergeometric function 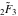 : :
Differential equations
The Airy functions 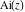 and and 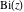 appeared as special solutions of the simple-looking linear second-order differential equation: appeared as special solutions of the simple-looking linear second-order differential equation:
where 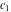 and and 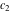 are arbitrary constants.
Additional restrictions on are arbitrary constants.
Additional restrictions on 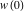 and and 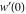 lead to corresponding Airy functions: lead to corresponding Airy functions:
Similar properties are valid for derivatives of Airy functions:
Applications of Airy functions
Applications of Airy functions include quantum mechanics of linear potential, electrodynamics, electromagnetism, combinatorics, analysis of the algorithmic complexity, optical theory of the rainbow, solid state physics, radiative transfer, and semiconductors in electric fields.
|