|
Entering the complex plane 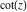 in the in the  near the real axis. Here the complex variable near the real axis. Here the complex variable 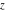 is expressed as is expressed as 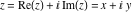 . The red surface is the real part of . The red surface is the real part of 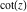 . The blue, semitransparent surface is the imaginary part of . The blue, semitransparent surface is the imaginary part of  . The pink tube is the function . The pink tube is the function 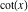 along the real axis. Along the real axis the real part of along the real axis. Along the real axis the real part of 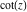 is oscillating and has first‐order poles at is oscillating and has first‐order poles at  . Going away from the real axis into the . Going away from the real axis into the  gives a function that approaches 0 exponentially. Along the real axis, the imaginary part of gives a function that approaches 0 exponentially. Along the real axis, the imaginary part of 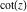 vanishes identically; going away from the real axis into the vanishes identically; going away from the real axis into the  gives a function that approaches gives a function that approaches  exponentially. exponentially.
Real part over the complex plane The real part of 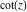 where where 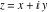 . The surface is colored according to the imaginary part. The right graphic is a contour plot of the scaled real part, meaning the height values of the left graphic translate into color values in the right graphic. Red is smallest and violet is largest. Along the real axis, the real part of . The surface is colored according to the imaginary part. The right graphic is a contour plot of the scaled real part, meaning the height values of the left graphic translate into color values in the right graphic. Red is smallest and violet is largest. Along the real axis, the real part of 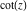 is oscillating; going away from the real axis into the is oscillating; going away from the real axis into the 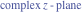 gives a function that approaches 0 exponentially. The first‐order poles at gives a function that approaches 0 exponentially. The first‐order poles at 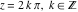 are clearly visible. are clearly visible.  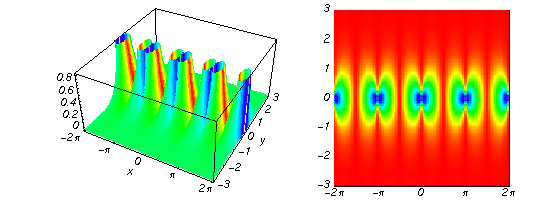 |
The absolute value of the real part of  where where 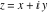 . The surface is colored according to the absolute value of the imaginary part. The right graphic is a contour plot of the scaled absolute value of the real part , meaning the height values of the left graphic translate into color values in the right graphic. Red is smallest and violet is largest. Along the real axis the absolute value of the real part of . The surface is colored according to the absolute value of the imaginary part. The right graphic is a contour plot of the scaled absolute value of the real part , meaning the height values of the left graphic translate into color values in the right graphic. Red is smallest and violet is largest. Along the real axis the absolute value of the real part of 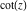 is oscillating; going away from the real axis into the is oscillating; going away from the real axis into the  gives a function that approaches 0 exponentially. The first‐order poles at gives a function that approaches 0 exponentially. The first‐order poles at 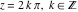 are clearly visible. are clearly visible. Imaginary part over the complex plane The imaginary part of 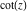 where where 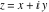 . The surface is colored according to the real part. The right graphic is a contour plot of the scaled imaginary part, meaning the height values of the left graphic translate into color values in the right graphic. Red is smallest and violet is largest. Along the real axis, the imaginary part of . The surface is colored according to the real part. The right graphic is a contour plot of the scaled imaginary part, meaning the height values of the left graphic translate into color values in the right graphic. Red is smallest and violet is largest. Along the real axis, the imaginary part of 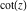 vanishes identically; going away from the real axis into the vanishes identically; going away from the real axis into the 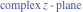 gives a function that approaches gives a function that approaches 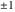 exponentially. The first‐order poles at exponentially. The first‐order poles at 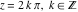 are clearly visible. are clearly visible.  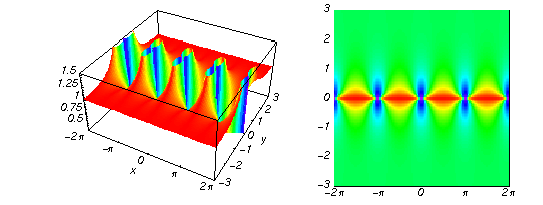 |
The absolute value of the imaginary part of 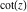 where where 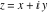 . The surface is colored according to the absolute value of the real part. The right graphic is a contour plot of the scaled absolute value of the imaginary part, meaning the height values of the left graphic translate into color values in the right graphic. Red is smallest and violet is largest. Along the real axis, the absolute value of the imaginary part of . The surface is colored according to the absolute value of the real part. The right graphic is a contour plot of the scaled absolute value of the imaginary part, meaning the height values of the left graphic translate into color values in the right graphic. Red is smallest and violet is largest. Along the real axis, the absolute value of the imaginary part of 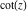 vanishes identically; going away from the real axis into the vanishes identically; going away from the real axis into the  gives a function that approaches gives a function that approaches 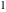 exponentially. The first‐order poles at exponentially. The first‐order poles at 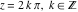 are clearly visible. are clearly visible. Absolute value part over the complex plane The absolute value of 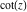 where where 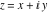 . The surface is colored according to the argument. The right graphic is a contour plot of the scaled absolute value, meaning the height values of the left graphic translate into color values in the right graphic. Red is smallest and violet is largest. Along the real axis . The surface is colored according to the argument. The right graphic is a contour plot of the scaled absolute value, meaning the height values of the left graphic translate into color values in the right graphic. Red is smallest and violet is largest. Along the real axis 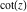 has poles; going away from the real axis gives a function that approaches 1 exponentially. The first‐order poles at has poles; going away from the real axis gives a function that approaches 1 exponentially. The first‐order poles at 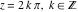 are clearly visible. are clearly visible. Argument over the complex plane The argument of 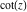 where where 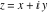 . The surface is colored according to the absolute value. The right graphic is a contour plot of the scaled argument, meaning the height values of the left graphic translate into color values in the right graphic. Red is smallest and violet is largest. . The surface is colored according to the absolute value. The right graphic is a contour plot of the scaled argument, meaning the height values of the left graphic translate into color values in the right graphic. Red is smallest and violet is largest. 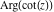 has lines of discontinuities over the has lines of discontinuities over the 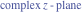 . . The square of the sine of the argument of 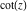 where where 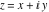 . For dominantly real values, the function values are near 0, and for dominantly imaginary values, the function values are near 1. The surface is colored according to the absolute value. The right graphic is a cyclically colored contour plot of the argument. Red represents arguments near . For dominantly real values, the function values are near 0, and for dominantly imaginary values, the function values are near 1. The surface is colored according to the absolute value. The right graphic is a cyclically colored contour plot of the argument. Red represents arguments near 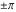 and light‐blue represents arguments near 0. and light‐blue represents arguments near 0. Zero-pole plot The logarithm of the absolute value of 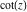 where where 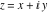 in the upper half plane. The surface is colored according to the square of the argument. In this plot zeros are easily visible as spikes extending downwards and poles and logarithmic singularities as spikes extending upwards. in the upper half plane. The surface is colored according to the square of the argument. In this plot zeros are easily visible as spikes extending downwards and poles and logarithmic singularities as spikes extending upwards. Real part over the complex plane near infinity The real part of 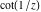 where where 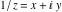 . The surface is colored according to the imaginary part.The right graphic is a contour plot of the scaled real part, meaning the height values of the left graphic translate into color values in the right graphic. Red is smallest and violet is largest. The essential singularity at . The surface is colored according to the imaginary part.The right graphic is a contour plot of the scaled real part, meaning the height values of the left graphic translate into color values in the right graphic. Red is smallest and violet is largest. The essential singularity at 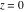 results in a complicated structure that cannot be resolved in graphically. results in a complicated structure that cannot be resolved in graphically. The absolute value of the real part of 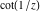 where where 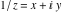 . The surface is colored according to the absolute value of the imaginary part. The right graphic is a contour plot of the scaled absolute value of the real part, meaning the height values of the left graphic translate into color values in the right graphic. Red is smallest and violet is largest. The essential singularity at . The surface is colored according to the absolute value of the imaginary part. The right graphic is a contour plot of the scaled absolute value of the real part, meaning the height values of the left graphic translate into color values in the right graphic. Red is smallest and violet is largest. The essential singularity at 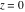 results in a complicated structure that cannot be resolved in graphically. results in a complicated structure that cannot be resolved in graphically. Imaginary part over the complex plane near infinity The imaginary part of 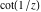 where where 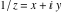 . The surface is colored according to the real part. The right graphic is a contour plot of the scaled imaginary part, meaning the height values of the left graphic translate into color values in the right graphic. Red is smallest and violet is largest. The essential singularity at . The surface is colored according to the real part. The right graphic is a contour plot of the scaled imaginary part, meaning the height values of the left graphic translate into color values in the right graphic. Red is smallest and violet is largest. The essential singularity at 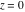 results in a complicated structure. The accumulation of poles near results in a complicated structure. The accumulation of poles near 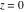 cannot be resolved graphically. cannot be resolved graphically. The absolute value of the imaginary part of 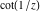 where where 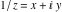 . The surface is colored according to the absolute value of the real part. The right graphic is a contour plot of the scaled absolute value of the imaginary part, meaning the height values of the left graphic translate into color values in the right graphic. Red is smallest and violet is largest. The essential singularity at . The surface is colored according to the absolute value of the real part. The right graphic is a contour plot of the scaled absolute value of the imaginary part, meaning the height values of the left graphic translate into color values in the right graphic. Red is smallest and violet is largest. The essential singularity at 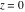 results in a complicated structure. The accumulation of poles near results in a complicated structure. The accumulation of poles near 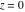 cannot be resolved graphically. cannot be resolved graphically. Absolute value part over the complex plane near infinity The absolute value of 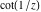 where where 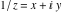 . The surface is colored according to the argument. The right graphic is a contour plot of the scaled absolute value, meaning the height values of the left graphic translate into color values in the right graphic. Red is smallest and violet is largest. The essential singularity at . The surface is colored according to the argument. The right graphic is a contour plot of the scaled absolute value, meaning the height values of the left graphic translate into color values in the right graphic. Red is smallest and violet is largest. The essential singularity at 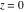 results in a complicated structure. The accumulation of poles near results in a complicated structure. The accumulation of poles near 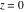 cannot be resolved graphically. cannot be resolved graphically. Argument over the complex plane near infinity The argument of 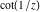 where where 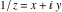 . The surface is colored according to the absolute value. The right graphic is a contour plot of the scaled argument, meaning the height values of the left graphic translate into color values in the right graphic. Red is smallest and violet is largest.The essential singularity at . The surface is colored according to the absolute value. The right graphic is a contour plot of the scaled argument, meaning the height values of the left graphic translate into color values in the right graphic. Red is smallest and violet is largest.The essential singularity at 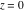 results in a complicated structure. The accumulation of poles near results in a complicated structure. The accumulation of poles near 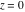 cannot be resolved graphically. cannot be resolved graphically. The square of the sine of the argument of 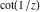 where where 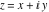 . For dominantly real values, the function values are near 0, and for dominantly imaginary values, the function values are near 1. The surface is colored according to the absolute value. The right graphic is a cyclically colored contour plot of the argument. Red represents arguments near . For dominantly real values, the function values are near 0, and for dominantly imaginary values, the function values are near 1. The surface is colored according to the absolute value. The right graphic is a cyclically colored contour plot of the argument. Red represents arguments near 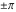 and light‐blue represents arguments near 0. and light‐blue represents arguments near 0. Zero-pole plot near infinity The logarithm of the absolute value of 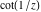 where where 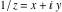 in the upper half‐plane. The surface is colored according to the square of the argument. In this plot zeros are easily visible as spikes extending downwards and poles and logarithmic singularities as spikes extending upwards. The essential singularity at in the upper half‐plane. The surface is colored according to the square of the argument. In this plot zeros are easily visible as spikes extending downwards and poles and logarithmic singularities as spikes extending upwards. The essential singularity at 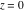 results in a complicated structure. The accumulation of poles near results in a complicated structure. The accumulation of poles near 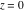 cannot be resolved graphically. cannot be resolved graphically.
|