|
The hyperbolic cosecant function 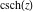 can be represented using more general mathematical functions. As the reciprocal to the hyperbolic sine function that is a particular case of the generalized hypergeometric, Bessel, Struve, and Mathieu functions, the hyperbolic cosecant function can also be represented as reciprocal to those special functions. Here are some examples: can be represented using more general mathematical functions. As the reciprocal to the hyperbolic sine function that is a particular case of the generalized hypergeometric, Bessel, Struve, and Mathieu functions, the hyperbolic cosecant function can also be represented as reciprocal to those special functions. Here are some examples:
But these representations are not very useful because they include complicated special functions in the denominators.
It is more interesting to write the hyperbolic cosecant function as particular cases of one special function. Such formulas take place for doubly periodic Jacobi elliptic functions that can degenerate into a hyperbolic cosecant function when their second parameter is equal to 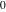 or or 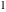 : :
|