Introduction to the Hyperbolic Cosecant Function in Mathematica

Overview

The following shows how the hyperbolic cosecant function is realized in Mathematica. Examples of evaluating Mathematica functions applied to various numeric and exact expressions that involve the hyperbolic cosecant function or return it are shown. These involve numeric and symbolic calculations and plots.

Notations

Mathematica forms of notations

Following Mathematica's general naming convention, function names in StandardForm are just the capitalized versions of their traditional mathematics names. This shows the hyperbolic cosecant function in StandardForm.

This shows the hyperbolic cosecant function in TraditionalForm.

Additional forms of notations

Mathematica also knows the most popular forms of notations for the hyperbolic cosecant function that are used in other programming languages. Here are three examples: CForm, TeXForm, and FortranForm.

Automatic evaluations and transformations

Evaluation for exact and machine-number values of arguments

For the exact argument , Mathematica returns an exact result.

For a machine‐number argument (numerical argument with a decimal point), a machine number is also returned.

The following inputs calculate 100‐digit approximations at and .

It is possible to calculate thousands of digits for the hyperbolic cosecant function in less than a second. The next input calculates 10000 digits for and analyzes the frequency of the digit in the resulting decimal number.

Here is a 50‐digit approximation to the hyperbolic cosecant function at the complex argument .

Mathematica automatically evaluates mathematical functions with machine precision, if the arguments of the function are numerical values and include machine‐number elements. Typically, only six digits after the decimal point are shown in the results. The remaining digits are suppressed, but can be displayed using the function InputForm.

Simplification of the argument

Mathematica knows the symmetry and periodicity of the the hyperbolic cosecant function. Here are some examples.

Mathematica automatically simplifies the composition of the direct and the inverse hyperbolic cosecant functions into its inner argument.

Mathematica also automatically simplifies the composition of the direct and any of the inverse hyperbolic functions into algebraic functions of the argument.

In cases where the argument has the structure or , and or with integer , the hyperbolic cosecant function can be automatically transformed into hyperbolic or trigonometric cosecant or secant functions.

Simplification of combinations of hyperbolic cosecant functions

Sometimes simple arithmetic operations containing the hyperbolic cosecant function can automatically generate other equal hyperbolic functions.

The hyperbolic cosecant function arising as special cases from more general functions

The hyperbolic cosecant function can be treated as a particular case of some more general special functions. For example, appears automatically from Bessel, Struve, Mathieu, Jacobi, hypergeometric, and Meijer functions or their reciprocals for appropriate parameters.

Equivalence transformations using specialized Mathematica functions

General remarks

Almost everybody prefers using instead of . Mathematica automatically transforms the second expression into the first one. The automatic application of transformation rules to mathematical expressions can give overly complicated results. Compact expressions like should not be automatically expanded into the more complicated expression . Mathematica has special functions that produce such expansions. Some are demonstrated in the next section.

TrigExpand

The function TrigExpand expands out trigonometric and hyperbolic functions. In more detail, it splits up sums and integer multiples that appear in the arguments of trigonometric and hyperbolic functions, and then expands out the products of trigonometric and hyperbolic functions into sums of powers, using trigonometric and hyperbolic identities where possible. Here are some examples.

TrigFactor

The function TrigFactor factors trigonometric and hyperbolic functions. In more detail, it splits up sums and integer multiples that appear in the arguments of trigonometric and hyperbolic functions, and then factors the resulting polynomials into trigonometric and hyperbolic functions, using trigonometric and hyperbolic identities where possible. Here are some examples.

TrigReduce

The function TrigReduce rewrites the products and powers of trigonometric and hyperbolic functions in terms of trigonometric and hyperbolic functions with combined arguments. In more detail, it typically yields a linear expression involving trigonometric and hyperbolic functions with more complicated arguments. TrigReduce is approximately opposite to TrigExpand and TrigFactor. Here are some examples.

TrigToExp

The function TrigToExp converts trigonometric and hyperbolic functions to exponentials. It tries, where possible, to give results that do not involve explicit complex numbers. Here are some examples.

ExpToTrig

The function ExpToTrig converts exponentials to trigonometric and hyperbolic functions. It is approximately opposite to TrigToExp. Here are some examples.

ComplexExpand

The function ComplexExpand expands expressions assuming that all the variables are real. The option TargetFunctions can be given as a list of functions from the set {Re, Im, Abs, Arg, Conjugate, Sign}. ComplexExpand will try to give results in terms of the specified functions. Here are some examples.

Simplify

The function Simplify performs a sequence of algebraic transformations on the expression, and returns the simplest form it finds. Here are some examples.

Here is a collection of hyperbolic identities. Each is written as a logical conjunction.

The function Simplify has the Assumption option. For example, Mathematica treats the periodicity of hyperbolic functions for the symbolic integer coefficient of .

Mathematica also knows that the composition of the inverse and direct hyperbolic functions produces the value of the inner argument under the corresponding restriction.

FunctionExpand (and Together)

While the hyperbolic cosecant function auto‐evaluates for simple fractions of , for more complicated cases it stays as a hyperbolic cosecant function to avoid the build up of large expressions. Using the function FunctionExpand, the hyperbolic cosecant function can sometimes be transformed into explicit radicals. Here are some examples.

If the denominator contains squares of integers other than 2, the results always contain complex numbers deeply inside the expression (meaning that the imaginary number appears unavoidably).

Here the function RootReduce is used to express the previous algebraic numbers as the roots of polynomial equations.

The function FunctionExpand also reduces hyperbolic expressions with compound arguments or compositions, including hyperbolic functions, to simpler ones. Here are some examples.

Applying Simplify to the last expression gives a more compact result.

FullSimplify

The function FullSimplify tries a wider range of transformations than Simplify and returns the simplest form it finds. Here are some examples that compare the results of applying these functions to the same expressions.

Operations under special Mathematica functions

Series expansions

Calculating the series expansion of a hyperbolic cosecant function to hundreds of terms can be done in seconds.

Mathematica comes with the add‐on package DiscreteMath`RSolve` that allows finding the general terms of the series for many functions. After loading this package, and using the package function SeriesTerm, the following 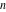term of can be evaluated.

This result can be verified by the following process.

Differentiation

Mathematica can evaluate derivatives of the hyperbolic cosecant function of an arbitrary positive integer order.

Indefinite integration

Mathematica can calculate a huge set of doable indefinite integrals that contain the hyperbolic cosecant function. The results can contain special functions. Here are some examples.

Definite integration

Mathematica can calculate wide classes of definite integrals that contain the hyperbolic cosecant function. Here are some examples.

Limit operation

Mathematica can calculate limits that contain the hyperbolic cosecant function. Here are some examples.

Solving equations

The next inputs solve two equations that contain the hyperbolic cosecant function. Because of the multivalued nature of the inverse hyperbolic cosecant function, a message is printed indicating that only some of the possible solutions are returned.

A complete solution of the previous equation can be obtained using the function Reduce.

Solving differential equations

Here is a linear first-order differential equation that is obeyed by the hyperbolic cosecant function.

Here is a nonlinear second-order differential equation that is obeyed by the hyperbolic cosecant function. Mathematica solves the differential equation as a rational function of . But it is straightforward to express the result as a hyperbolic cosecant function.

Plotting

Mathematica has built‐in functions for 2D and 3D graphics. Here are some examples.