|
The hyperbolic functions are particular cases of more general functions. Among these more general functions, four classes of special functions are of special relevance: Bessel, Jacobi, Mathieu, and hypergeometric functions.
For example, 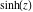 and and 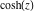 have the following representations through Bessel, Mathieu, and hypergeometric functions: have the following representations through Bessel, Mathieu, and hypergeometric functions:
All hyperbolic functions can be represented as degenerate cases of the corresponding doubly periodic Jacobi elliptic functions when their second parameter is equal to 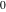 or or 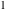 : :
Each of the six hyperbolic functions can be represented through the corresponding trigonometric function:
Each of the six hyperbolic functions is connected with a corresponding inverse hyperbolic function by two formulas. One direction can be expressed through a simple formula, but the other direction is much more complicated because of the multivalued nature of the inverse function:
Each of the six hyperbolic functions can be represented through any other function as a rational function of that function with a linear argument. For example, the hyperbolic sine can be representative as a group‐defining function because the other five functions can be expressed as:
All six hyperbolic functions can be transformed into any other function of the group of hyperbolic functions if the argument  is replaced by is replaced by 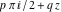 with with 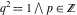 : :
|