|
For real values of argument 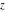 , the values of all the hyperbolic functions are real (or infinity). , the values of all the hyperbolic functions are real (or infinity).
In the points 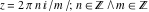 , the values of the hyperbolic functions are algebraic. In several cases, they can even be rational numbers, , the values of the hyperbolic functions are algebraic. In several cases, they can even be rational numbers, 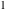 , or , or  (e.g. (e.g. 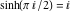 , , 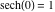 , or , or 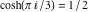 ). They can be expressed using only square roots if ). They can be expressed using only square roots if 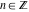 and and 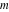 is a product of a power of 2 and distinct Fermat primes {3, 5, 17, 257, …}. is a product of a power of 2 and distinct Fermat primes {3, 5, 17, 257, …}.
All hyperbolic functions has rather simple values for arguments 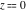 and and 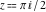 : :
All hyperbolic functions are defined for all complex values of 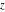 , and they are analytical functions of , and they are analytical functions of 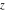 over the whole complex over the whole complex 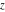 ‐plane and do not have branch cuts or branch points. The two functions ‐plane and do not have branch cuts or branch points. The two functions 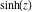 and and 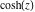 are entire functions with an essential singular point at are entire functions with an essential singular point at 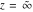 . All other hyperbolic functions are meromorphic functions with simple poles at points . All other hyperbolic functions are meromorphic functions with simple poles at points  (for (for 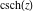 and and 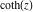 ) and at points ) and at points 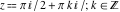 (for (for  and and 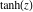 ). ).
All hyperbolic functions are periodic functions with a real period (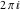 or or 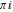 ): ):
All hyperbolic functions have parity (either odd or even) and mirror symmetry:
The derivatives of all hyperbolic functions have simple representations that can be expressed through other hyperbolic functions:
The solutions of the simplest second‐order linear ordinary differential equation with constant coefficients can be represented through 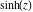 and and 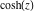 . The other hyperbolic functions satisfy first‐order nonlinear differential equations: . The other hyperbolic functions satisfy first‐order nonlinear differential equations:
All six hyperbolic functions satisfy first‐order nonlinear differential equations:
|