|
Trigonometric functions are intimately related to triangle geometry. Functions like sine and cosine are often introduced as edge lengths of right‐angled triangles. Hyperbolic functions occur in the theory of triangles in hyperbolic spaces.
Lobachevsky (1829) and J. Bolyai (1832) independently recognized that Euclid's fifth postulate—saying that for a given line and a point not on the line, there is exactly one line parallel to the first—might be changed and still be a consistent geometry. In the hyperbolic geometry it is allowable for more than one line to be parallel to the first (meaning that the parallel lines will never meet the first, however far they are extended). Translated into triangles, this means that the sum of the three angles is always less than 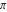 . .
A particularly nice representation of the hyperbolic geometry can be realized in the unit disk of complex numbers (the Poincaré disk model). In this model, points are complex numbers in the unit disk, and the lines are either arcs of circles that meet the boundary of the unit circle orthogonal or diameters of the unit circle.
The distance  between two points (meaning complex numbers) between two points (meaning complex numbers) 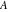 and and 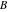 in the Poincaré disk is: in the Poincaré disk is:
The attractive feature of the Poincaré disk model is that the hyperbolic angles agree with the Euclidean angles. Formally, the angle 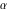 at a point at a point 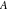 of two hyperbolic lines of two hyperbolic lines 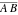 and and 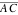 is described by the formula: is described by the formula:
In the following, the values of the three angles of an hyperbolic triangle at the vertices 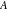 , ,  , and , and 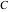 are denoted through are denoted through  , , 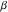 , and , and  . The hyperbolic length of the three edges opposite to the angles are denoted . The hyperbolic length of the three edges opposite to the angles are denoted 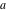 , , 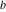 , and , and 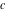 . .
The cosine rule and the second cosine rule for hyperbolic triangles are:
The sine rule for hyperbolic triangles is:
For a right‐angle triangle, the hyperbolic version of the Pythagorean theorem follows from the preceding formulas (the right angle is taken at vertex 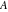 ): ):
Using the series expansion 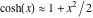 at small scales the hyperbolic geometry is approximated by the familar Euclidean geometry. The cosine formulas and the sine formulas for hyperbolic triangles with a right angle at vertex at small scales the hyperbolic geometry is approximated by the familar Euclidean geometry. The cosine formulas and the sine formulas for hyperbolic triangles with a right angle at vertex 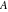 become: become:
The inscribed circle has the radius:
The circumscribed circle has the radius:
As rational functions of the exponential function, the hyperbolic functions appear virtually everywhere in quantitative sciences. It is impossible to list their numerous applications in teaching, science, engineering, and art.
|