|
Introduction to the incomplete elliptic integrals
General
Elliptic integrals were encountered in the work of J. Wallis (1655–1659) who investigated the integral in modern notation:
Broader interest in such integrals was stimulated by the appearance and development of integral calculus in the 18th and 19th centuries. Mathematicians found that integrals, containing quadratic polynomials under a square root of the form:
can be evaluated through elementary functions by formulas such as:
But these early mathematicians could not find simple formulas for similar integrals containing higher-degree polynomials:
Many important applications of these integrals were found at that time. The problem of evaluating such integrals was converted into the problem of evaluating only three basic integrals. They were later denoted by their special notation and named the incomplete elliptic integrals of the first, second, and third kinds—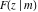 , , 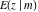 , and , and 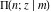 (A. M. Legendre): (A. M. Legendre):
The corresponding definite integrals (for 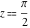 ) were named the complete elliptic integrals of the first, second, and third kinds denoted by the symbols ) were named the complete elliptic integrals of the first, second, and third kinds denoted by the symbols 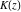 , , 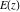 , and , and 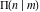 : :
These integrals were extensively studied for another important reason—development of the theory of the double periodic functions. These functions were called elliptic functions. The elliptic integrals and elliptic functions were studied simultaneously on several occasions throughout history and a deep connection exists between these two areas of mathematics. The following chronology reflects the main steps in building the theory of elliptic integrals.
L. Euler (1733, 1757, 1763, 1766) derived the addition theorem for the incomplete elliptic integrals 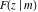 , and , and 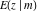 . .
J.‐L. Lagrange (1783) and especially A. M. Legendre (1793, 1811, 1825–1828) devoted a lot of attention to the study of the different properties of those two elliptic integrals. C. F. Gauss (1799, 1818) also used these integrals during his research.
Simultaneously, A. M. Legendre (1811) introduced the incomplete elliptic integral of the third kind and the complete versions of all three elliptic integrals.
C. G. J. Jacobi (1827–1829) introduced inverse functons of the elliptic integrals 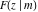 and and 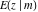 , which led him to build the theory of elliptic functions. In 1829, C. G. J. Jacobi defined the following function: , which led him to build the theory of elliptic functions. In 1829, C. G. J. Jacobi defined the following function:
which was later called the Jacobi zeta function. J. Liouville (1840) also studied elliptic integrals 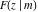 and and 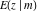 . .
N. H. Abel, independently from C. G. J. Jacobi, got some of his results and studied the so-called hyperelliptic and Abelian integrals.
Definitions of incomplete elliptic integrals
The incomplete elliptic integral of the first kind 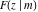 , incomplete elliptic integral of the second kind , incomplete elliptic integral of the second kind 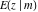 , incomplete elliptic integral of the third kind , incomplete elliptic integral of the third kind 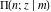 , and Jacobi zeta function , and Jacobi zeta function 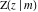 are defined by the following formulas: are defined by the following formulas:
The previous functions are called incomplete elliptic integrals.
A quick look at the incomplete elliptic integrals Here is a quick look at the graphics for the incomplete elliptic integrals along the real axis.
Connections within the group of incomplete elliptic integrals and with other function groups
Representations through more general functions
The incomplete elliptic integrals 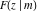 , , 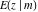 , , 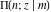 , and , and 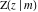 can be represented through more general functions. Through the hypergeometric Appell can be represented through more general functions. Through the hypergeometric Appell 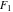 function of two variables: function of two variables:
Through the generalized hypergeometric function of two variables:
Through elliptic theta functions, for example:
Through inverse Jacobi elliptic functions, for example:
Through Weierstrass elliptic functions and inverse elliptic nome 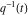 , for example: , for example:
Through some elliptic‐type functions, for example:
Representations through related functions
The incomplete elliptic integrals 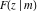 , ,  , , 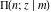 , and , and 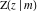 can be represented through some related functions, for example: can be represented through some related functions, for example:
Relations to inverse functions
The incomplete elliptic integral 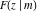 is related to the Jacobi amplitude by the following formulas, which demonstrate that the Jacobi amplitude is within a restricted domain, the inverse function of elliptic integral is related to the Jacobi amplitude by the following formulas, which demonstrate that the Jacobi amplitude is within a restricted domain, the inverse function of elliptic integral 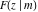 : :
Representations through other incomplete elliptic integrals
The incomplete elliptic integrals  , , 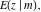 and and 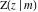 can be represented through incomplete elliptic integral can be represented through incomplete elliptic integral 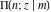 by the following formulas: by the following formulas:
The best-known properties and formulas for incomplete elliptic integrals
Simple values at zero
The incomplete elliptic integrals 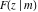 , , 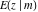 , , 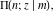 and and 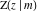 are equal to are equal to 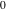 at the origin points: at the origin points:
Specific values
The incomplete elliptic integrals 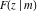 , , 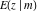 , , 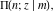 and and 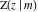 for particular argument values can be evaluated in closed forms, for example: for particular argument values can be evaluated in closed forms, for example:
At infinities, the incomplete elliptic integrals 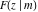 , , 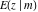 , , 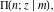 and and  have the following values: have the following values:
Analyticity
The incomplete elliptic integrals 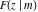 , , 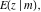 and and 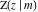 are analytical functions of are analytical functions of 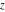 and and 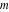 , which are defined over , which are defined over 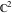 .
The incomplete elliptic integral .
The incomplete elliptic integral 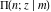 is an analytical function of is an analytical function of 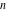 , , 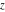 , and , and 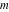 , which is defined over , which is defined over 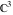 . .
Poles and essential singularities
The incomplete elliptic integrals 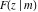 , , 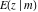 , , 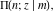 and and 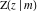 do not have poles and essential singularities with respect to their variables. do not have poles and essential singularities with respect to their variables.
Branch points and branch cuts
For fixed 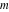 , the functions , the functions  , , 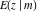 , and , and 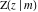 have an infinite number of branch points at have an infinite number of branch points at 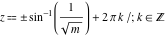 and and 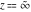 .
They have complicated branch cut. .
They have complicated branch cut.
For fixed 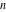 , , 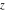 , the function , the function 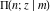 has two branch points at has two branch points at 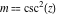 and and 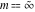 . For fixed . For fixed 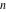 , , 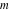 , the function , the function  has an infinite number of branch points at has an infinite number of branch points at 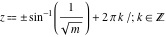 , , 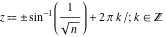 and and 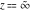 . For fixed . For fixed 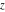 , , 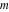 , the function , the function 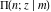 does not have branch points. does not have branch points.
Periodicity
The incomplete elliptic integrals 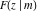 , , 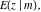 and and 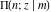 are quasi‐periodic functions with respect to are quasi‐periodic functions with respect to 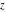 : :
The incomplete elliptic integral 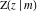 is a periodic function with respect to is a periodic function with respect to 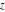 with period with period  : :
Parity and symmetry
The incomplete elliptic integrals 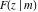 , , 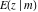 , , 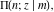 and and 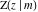 have mirror symmetry: have mirror symmetry:
The incomplete elliptic integrals 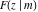 , , 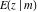 , , 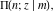 and and 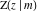 are odd functions with respect to are odd functions with respect to 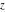 . .
Series representations
The incomplete elliptic integral 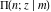 has the following series expansions at the point has the following series expansions at the point 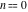 : :
The incomplete elliptic integrals 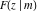 , , 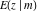 , , 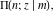 and and 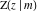 have the following series expansions at the point have the following series expansions at the point 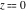 : :
The incomplete elliptic integrals 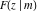 , ,  , , 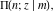 and and 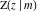 have the following series expansions at the point have the following series expansions at the point 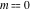 : :
The incomplete elliptic integrals 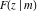 , , 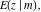 and and 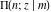 have the following series expansions at the point have the following series expansions at the point 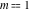 : :
Other series representations
The incomplete elliptic integrals 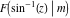 and and 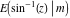 have the following series expansions at the point have the following series expansions at the point 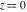 : :
The incomplete elliptic integrals 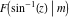 and and 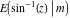 have the following series expansions at the point have the following series expansions at the point 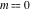 : :
The incomplete elliptic integrals 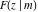 , ,  , , 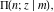 and and 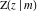 can be represented through different kinds of series, for example: can be represented through different kinds of series, for example:
where 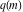 is an elliptic nome and is an elliptic nome and 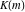 is a complete elliptic integral. is a complete elliptic integral.
Integral representations
The incomplete elliptic integrals 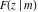 , , 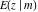 , ,  and and 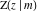 have the following integral representations: have the following integral representations:
Transformations
The incomplete elliptic integrals 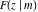 , , 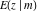 , , 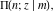 and and 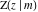 with linear arguments can sometimes be simplified, for example: with linear arguments can sometimes be simplified, for example:
In some cases, simplification can be realized for more complicated arguments, for example:
Sums of the incomplete elliptic integrals 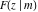 , , 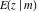 , , 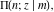 and and 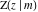 with different values with different values 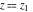 and and 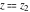 can be evaluated by the following summation formulas: can be evaluated by the following summation formulas:
Identities
The incomplete elliptic integrals 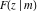 , , 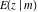 , , 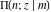 , and , and 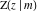 satisfy numerous identities, for example: satisfy numerous identities, for example:
Representations of derivatives
The first derivative of the incomplete elliptic integral 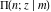 with respect to variable with respect to variable 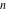 has the following representation: has the following representation:
The previous formula can be generalized to the  derivative: derivative:
The first derivatives of the incomplete elliptic integrals 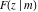 , , 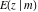 , , 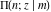 , and , and 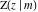 with respect to variable with respect to variable 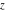 have the following simple representations: have the following simple representations:
The previous formulas can be generalized to the arbitrary-order symbolic derivatives:
The first derivatives of the incomplete elliptic integrals 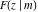 , ,  , , 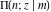 and and 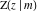 with respect to variable with respect to variable 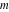 have the following representations: have the following representations:
The previous formulas can be generalized to the arbitrary-order symbolic derivatives:
Integration
The indefinite integral of the incomplete elliptic integral 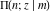 with respect to variable with respect to variable 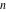 has the following representation through the infinite series that includes the Appell has the following representation through the infinite series that includes the Appell 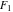 hypergeometric function of two variables: hypergeometric function of two variables:
The indefinite integrals of all incomplete elliptic integrals 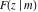 , , 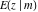 , , 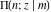 , and , and 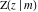 with respect to variable with respect to variable 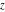 have the following representations: have the following representations:
The indefinite integrals of incomplete elliptic integrals  , , 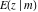 , and , and 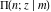 with respect to variable with respect to variable 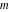 can be expressed in closed forms by the following formulas: can be expressed in closed forms by the following formulas:
Differential equations
The incomplete elliptic integrals 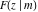 , ,  , , 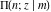 , and , and 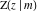 satisfy the following differential equations: satisfy the following differential equations:
Applications of incomplete elliptic integrals
Applications of incomplete elliptic integrals include geometry, physics, mechanics, electrodynamics, statistical mechanics, astronomy, geodesy, geodesics on conics, and magnetic field calculations.
|