|
Definitions of gamma functions
The gamma function 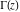 , the incomplete gamma function , the incomplete gamma function 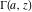 , the generalized incomplete gamma function , the generalized incomplete gamma function 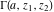 , the regularized incomplete gamma function , the regularized incomplete gamma function 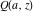 , the generalized regularized incomplete gamma function , the generalized regularized incomplete gamma function 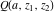 , the log‐gamma function (almost equal to the logarithm of the gamma function) , the log‐gamma function (almost equal to the logarithm of the gamma function)  , the inverse of the regularized incomplete gamma function , the inverse of the regularized incomplete gamma function 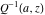 , and the inverse of the generalized regularized incomplete gamma function , and the inverse of the generalized regularized incomplete gamma function 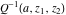 are defined by the following formulas: are defined by the following formulas:
The function
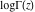
is equivalent to
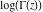
as a multivalued analytic function, except that it is conventionally defined with a different branch cut structure and principal sheet. The function
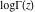
allows a concise formulation of many identities related to the Riemann zeta function
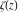
:
The previous functions comprise the interconnected group called the gamma functions.
Instead of the first three previous classical definitions using definite integrals, the other equivalent definitions with infinite series can be used.
|