|
The Fibonacci and Lucas numbers 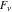 and and 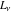 have the following values at zero and infinity: have the following values at zero and infinity:
The Fibonacci and Lucas numbers 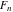 and and 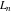 with integer argument with integer argument  can be represented by the following formulas: can be represented by the following formulas:
For the cases of integer arguments 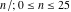 , the values of the Fibonacci and Lucas numbers , the values of the Fibonacci and Lucas numbers 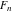 and and 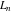 can be described by the following table: can be described by the following table:
The Fibonacci and Lucas numbers 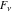 and and 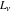 are entire analytical functions of are entire analytical functions of 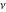 that are defined over the whole complex that are defined over the whole complex 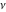 -plane: -plane:
The Fibonacci and Lucas numbers 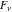 and and 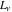 do not have periodicity. do not have periodicity.
The Fibonacci and Lucas numbers 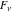 and and 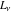 generically do not have parity, but they have mirror symmetry: generically do not have parity, but they have mirror symmetry:
The Fibonacci and Lucas numbers 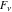 and and 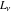 have only the singular point have only the singular point  . It is an essential singular point. . It is an essential singular point.
The Fibonacci and Lucas numbers 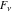 and and 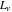 do not have branch points and branch cuts over the complex do not have branch points and branch cuts over the complex  -plane. -plane.
The Fibonacci and Lucas numbers 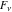 and and 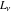 have the following series expansions (which converge in the whole complex have the following series expansions (which converge in the whole complex 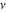 -plane): -plane):
The asymptotic behavior of the Fibonacci and Lucas numbers 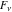 and and 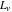 is described by the following formulas: is described by the following formulas:
The Fibonacci and Lucas numbers 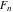 and and 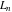 for integer nonnegative for integer nonnegative 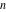 can be represented through the following sums involving binomials: can be represented through the following sums involving binomials:
The Fibonacci and Lucas numbers 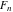 and and 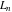 have the following integral representations on the real axis: have the following integral representations on the real axis:
The Fibonacci and Lucas numbers 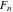 and and 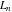 can be represented as the coefficients of the series of the corresponding generating functions: can be represented as the coefficients of the series of the corresponding generating functions:
The Fibonacci and Lucas numbers 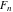 and and 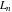 satisfy numerous addition formulas: satisfy numerous addition formulas:
The Fibonacci and Lucas numbers 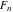 and and 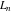 satisfy numerous identities, for example the following multiple argument formulas: satisfy numerous identities, for example the following multiple argument formulas:
The Fibonacci and Lucas numbers 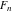 and and 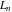 satisfy numerous identities for products and powers: satisfy numerous identities for products and powers:
The Fibonacci and Lucas numbers 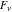 and and 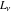 are solutions of the following simple difference equation with constant coefficients: are solutions of the following simple difference equation with constant coefficients:
The Fibonacci and Lucas numbers 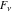 and and 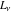 satisfy numerous recurrence identities: satisfy numerous recurrence identities:
Other identities for Fibonacci and Lucas numbers 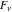 and and 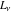 are just functional identities: are just functional identities:
The Fibonacci and Lucas numbers 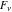 and and 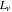 have the following complex characteristics for complex arguments: have the following complex characteristics for complex arguments:
The Fibonacci and Lucas numbers 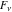 and and 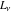 have the following representations for derivatives of the first and have the following representations for derivatives of the first and 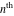 orders or the arbitrary fractional order orders or the arbitrary fractional order 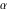 : :
The Fibonacci and Lucas numbers 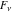 and and 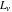 satisfy the following third-order linear differential equation: satisfy the following third-order linear differential equation:
where 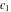 , , 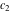 , and , and 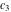 are arbitrary constants. are arbitrary constants.
Some indefinite integrals for Fibonacci and Lucas numbers 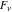 and and 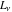 can be evaluated as follows: can be evaluated as follows:
Laplace transforms 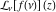 of the Fibonacci and Lucas numbers of the Fibonacci and Lucas numbers 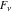 and and 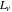 can be represented by the following formulas: can be represented by the following formulas:
There exist many formulas for finite summation of Fibonacci and Lucas numbers, for example:
Here are some corresponding infinite sums:
And here are some multiple sums:
Some formulas including limit operations with Fibonacci and Lucas numbers 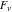 and and  take on symmetrical forms: take on symmetrical forms:
The Fibonacci numbers 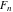 can be obtained from the evaluation of some determinates, for example: can be obtained from the evaluation of some determinates, for example:
|