|
Introduction to partitions
General
Interest in partitions appeared in the 17th century when G. W. Leibniz (1669) investigated the number of ways a given positive integer can be decomposed into a sum of smaller integers. Later, L. Euler (1740) also used partitions in his work. But extensive investigation of partitions began in the 20th century with the works of S. Ramanujan (1917) and G. H. Hardy. In particular G. H. Hardy (1920) introduced the notations  and and  to represent the two most commonly used types of parititions. to represent the two most commonly used types of parititions.
Definitions of partitions
The partition functions discussed here include two basic functions that describe the structure of integer numbers—the number of unrestricted partitions of an integer (partitions P) 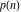 , and the number of partitions of an integer into distinct parts (partitions Q) , and the number of partitions of an integer into distinct parts (partitions Q) 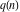 . .
Partitions P
For nonnegative integer 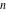 , the function , the function 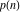 is the number of unrestricted partitions of the positive integer is the number of unrestricted partitions of the positive integer 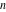 into a sum of strictly positive numbers that add up to into a sum of strictly positive numbers that add up to 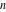 independent of the order, when repetitions are allowed. independent of the order, when repetitions are allowed.
The function  can be described by the following formulas: can be described by the following formulas:
where 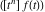 (with (with 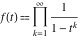 ) is the coefficient of the ) is the coefficient of the 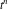 term in the series expansion around term in the series expansion around 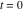 of the function of the function 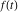 : : 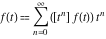 . .
Example: There are seven possible ways to express 5 as a sum of nonnegative integers: 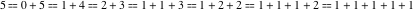 . For this reason . For this reason 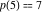 . .
Partitions Q
For nonnegative integer 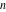 , the function , the function 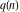 is the number of restricted partitions of the positive integer is the number of restricted partitions of the positive integer 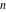 into a sum of distinct positive numbers that add up to into a sum of distinct positive numbers that add up to 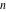 when order does not matter and repetitions are not allowed. when order does not matter and repetitions are not allowed.
The function  can be described by the following formulas: can be described by the following formulas:
where 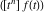 (with (with 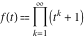 ) is the coefficient of the ) is the coefficient of the 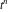 term in the series expansion around term in the series expansion around 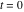 of the function of the function 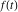 : : 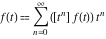 . .
Example: There are three possible ways to express 5 as a sum of nonnegative integers without repetitions: 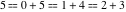 . For this reason . For this reason 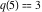 . .
Connections within the group of the partitions and with other function groups
Representations through related functions
The partition functions 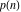 and and 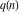 are connected by the following formula: are connected by the following formula:
The best-known properties and formulas of partitions
Simple values at zero and infinity
The partition functions 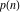 and and 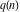 are defined for zero and infinity values of argument are defined for zero and infinity values of argument 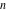 by the following rules: by the following rules:
Specific values for specialized variables
The following table represents the values of the partitions 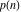 and and 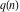 for for 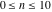 and some powers of 10: and some powers of 10:
Analyticity
The partition functions 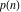 and and 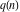 are non‐analytical functions that are defined only for integers. are non‐analytical functions that are defined only for integers.
Periodicity
The partition functions 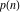 and and 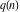 do not have periodicity. do not have periodicity.
Parity and symmetry
The partition functions 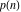 and and 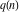 do not have symmetry. do not have symmetry.
Series representations
The partition functions 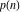 and and 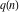 have the following series representations: have the following series representations:
where 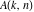 is a special case of a generalized Kloosterman sum: is a special case of a generalized Kloosterman sum:
Asymptotic series expansions
The partition functions 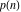 and and 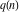 have the following asymptotic series expansions: have the following asymptotic series expansions:
Generating functions
The partition functions 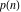 and and 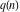 can be represented as the coefficients of their generating functions: can be represented as the coefficients of their generating functions:
where 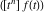 is the coefficient of the is the coefficient of the 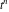 term in the series expansion around term in the series expansion around 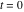 of the function of the function 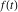 , , 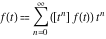 . .
Identities
The partition functions 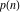 and and 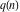 satisfy numerous identities, for example: satisfy numerous identities, for example:
Complex characteristics
As real valued functions, the partitions 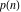 and and 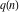 have the following complex characteristics: have the following complex characteristics:
Summation
There exist just a few formulas including finite and infinite summation of partitions, for example:
Inequalities
The partitions 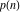 and and 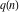 satisfy various inequalities, for example: satisfy various inequalities, for example:
Congruence properties
The 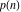 partitions have the following congruence properties: partitions have the following congruence properties:
Zeros
The 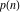 and and 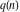 partitions have the following unique zeros: partitions have the following unique zeros:
Applications of partitions
Partitions are used in number theory and other fields of mathematics.
|