|
2D plots along the axes and unit circle On the real axis The function 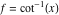 along the real axis. along the real axis. 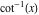 is real‐valued for all real arguments. At is real‐valued for all real arguments. At 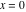 , the function , the function 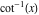 has a discontinuity. has a discontinuity. The function 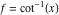 along the real axis. The imaginary part vanishes identically on the real axis. The left graphic shows along the real axis. The imaginary part vanishes identically on the real axis. The left graphic shows 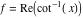 and the right graphic shows and the right graphic shows 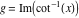 . . The absolute value and the argument of 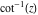 along the real axis. The left graphic shows along the real axis. The left graphic shows 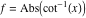 and the right graphic shows and the right graphic shows 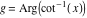 . The argument is piecewise constant. . The argument is piecewise constant. On the real axis at infinity The function 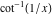 along the real axis. The left graphic shows along the real axis. The left graphic shows 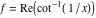 and the right graphic shows and the right graphic shows 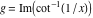 . . The absolute value and the argument of 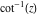 along the real axis. The left graphic shows along the real axis. The left graphic shows 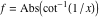 and the right graphic shows and the right graphic shows 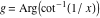 . The argument is piecewise constant. . The argument is piecewise constant. On the imaginary axes The real part and the imaginary part of 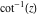 along the imaginary axis. The left graphic shows along the imaginary axis. The left graphic shows 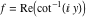 and the right graphic shows and the right graphic shows 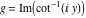 . Along the imaginary axis, . Along the imaginary axis, 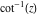 is complex valued with a piecewise constant real part, and the imaginary part is asymtotically a decreasing function. At the points is complex valued with a piecewise constant real part, and the imaginary part is asymtotically a decreasing function. At the points 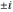 , the function , the function 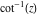 has logarithmic singularities. has logarithmic singularities. The absolute value and the argument of 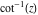 along the imaginary axis. The left graphic shows along the imaginary axis. The left graphic shows  and the right graphic shows and the right graphic shows 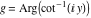 . Because . Because 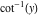 is purely imaginary outside the interval is purely imaginary outside the interval 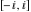 , the argument is constant there. , the argument is constant there. On the imaginary axis at infinity The function 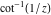 along the imaginary axis. The left graphic shows along the imaginary axis. The left graphic shows 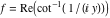 and the right graphic shows and the right graphic shows 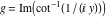 . At . At 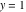 , the function , the function 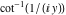 has logarithmic singularities. has logarithmic singularities. The absolute value and the argument of 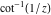 along the imaginary axis. The left graphic shows along the imaginary axis. The left graphic shows 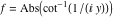 and the right graphic shows and the right graphic shows  . . On the unit circle The real part and the imaginary part of 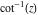 on the unit circle. The left graphic shows on the unit circle. The left graphic shows 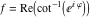 and the right graphic shows and the right graphic shows 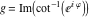 . Interestingly, the real part is piecewise constant with value . Interestingly, the real part is piecewise constant with value 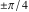 . . The absolute value and the argument of 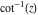 on the unit circle. The left graphic shows on the unit circle. The left graphic shows 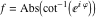 and the right graphic shows and the right graphic shows 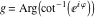 . .
|