|
3D plots over the complex plane Entering the complex plane  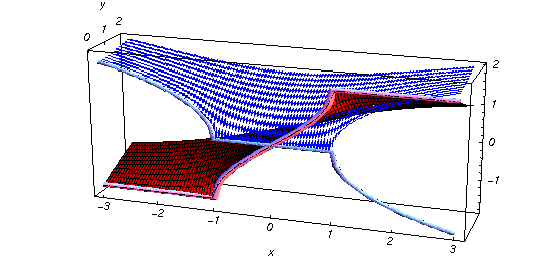 |
  |
Upper picture: 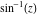 in the upper half of the in the upper half of the  near the real axis viewed from the lower half‐plane.
Lower picture: near the real axis viewed from the lower half‐plane.
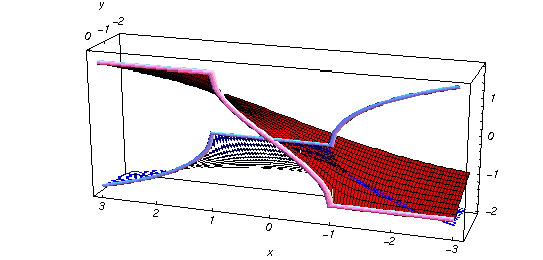
Lower picture: 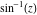 in the lower half of the in the lower half of the  near the real axis viewed from the upper half‐plane.
Here the complex variable near the real axis viewed from the upper half‐plane.
Here the complex variable 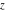 is expressed as is expressed as 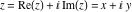 . The red surface is the real part of . The red surface is the real part of 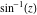 . The blue, semitransparent surface is the imaginary part of . The blue, semitransparent surface is the imaginary part of  . The pink tube is the real part of the function . The pink tube is the real part of the function 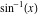 along the real axis, and the skyblue tube is the imaginary part of the function along the real axis, and the skyblue tube is the imaginary part of the function 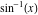 along the real axis. Along the real axis outside the interval along the real axis. Along the real axis outside the interval 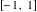 , the real part of , the real part of 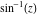 is constant; going away from the real axis into the upper half of the is constant; going away from the real axis into the upper half of the  gives a function that approaches 0 as gives a function that approaches 0 as 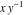 . Along the real axis, the imaginary part of . Along the real axis, the imaginary part of 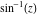 vanishes identically in the interval vanishes identically in the interval 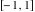 ; going away from the real axis into the ; going away from the real axis into the  is a function that approaches is a function that approaches 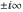 logarithmically. The real part is a continuous function, but not a continuously differentiable function, and the imaginary part is discontinuous along the branch cuts logarithmically. The real part is a continuous function, but not a continuously differentiable function, and the imaginary part is discontinuous along the branch cuts 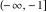 and and 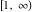 . The imaginary part has upper lip continuity in the left half plane and lower lip continuity in the right half plane. . The imaginary part has upper lip continuity in the left half plane and lower lip continuity in the right half plane. Branch cuts The real part and the imaginary part of 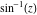 over the over the 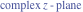 . The left graphic shows . The left graphic shows 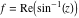 and the right graphic shows and the right graphic shows 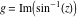 . Along the intervals . Along the intervals 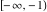 , , 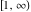 the function the function 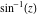 has branch cuts. The imaginary part has discontinuities along the branch cuts. The branch points at has branch cuts. The imaginary part has discontinuities along the branch cuts. The branch points at 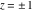 are of square root type. The viewpoint is from the lower half‐plane. are of square root type. The viewpoint is from the lower half‐plane. The real part and the imaginary part of  over the over the  . The left graphic shows . The left graphic shows 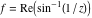 and the right graphic shows and the right graphic shows 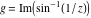 . The logarithmic singularity at . The logarithmic singularity at 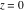 and the corresponding branch cut are visible. The viewpoint is from the upper half‐plane. and the corresponding branch cut are visible. The viewpoint is from the upper half‐plane. The branch cuts of the real part and the imaginary part of 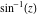 over the over the 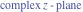 . The left graphic shows . The left graphic shows 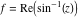 and the right graphic shows and the right graphic shows 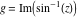 . The red and blue vertical surfaces connect points from the immediate lower and upper neighborhood of the branch cuts. The branch points at . The red and blue vertical surfaces connect points from the immediate lower and upper neighborhood of the branch cuts. The branch points at 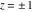 are of square root type. Only the imaginary part shows discontinuities due to the branch cuts. The viewpoint is from the lower half‐plane. are of square root type. Only the imaginary part shows discontinuities due to the branch cuts. The viewpoint is from the lower half‐plane. The branch cuts of the real part and the imaginary part of 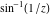 over the over the  . The left graphic shows . The left graphic shows 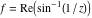 and the right graphic shows and the right graphic shows 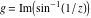 . The red and blue vertical surfaces connect points from the immediate lower and upper neighborhood of the branch cuts. The logarithmic singularity at . The red and blue vertical surfaces connect points from the immediate lower and upper neighborhood of the branch cuts. The logarithmic singularity at 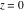 and the corresponding branch cut are visible. The viewpoint is from the upper half‐plane. and the corresponding branch cut are visible. The viewpoint is from the upper half‐plane. Real part over the complex plane The real part of  where where 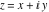 . The surface is colored according to the imaginary part. The right graphic is a contour plot of the scaled real part, meaning, the height values of the left graphic translate into color values in the right graphic. Red is smallest and violet is largest. Along the real axis outside the interval . The surface is colored according to the imaginary part. The right graphic is a contour plot of the scaled real part, meaning, the height values of the left graphic translate into color values in the right graphic. Red is smallest and violet is largest. Along the real axis outside the interval 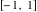 , the real part of , the real part of 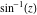 is constant; going away from the real axis into the upper half of the is constant; going away from the real axis into the upper half of the  gives a function that approaches 0 as gives a function that approaches 0 as 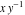 . . The absolute value of the real part of 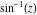 where where  . The surface is colored according to the absolute value of the imaginary part. The right graphic is a contour plot of the scaled absolute value of the real part, meaning the height values of the left graphic translate into color values in the right graphic. Red is smallest and violet is largest. Along the real axis outside the interval . The surface is colored according to the absolute value of the imaginary part. The right graphic is a contour plot of the scaled absolute value of the real part, meaning the height values of the left graphic translate into color values in the right graphic. Red is smallest and violet is largest. Along the real axis outside the interval 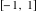 , the real part of , the real part of 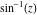 is constant; going away from the real axis into the upper half of the is constant; going away from the real axis into the upper half of the 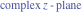 gives a function that approaches 0 as gives a function that approaches 0 as 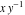 . . Imaginary part over the complex plane The imaginary part of 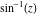 where where 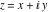 . The surface is colored according to the real part. The right graphic is a contour plot of the scaled imaginary part, meaning the height values of the left graphic translate into color values in the right graphic. Red is smallest and violet is largest. Along the real axis, the imaginary part of . The surface is colored according to the real part. The right graphic is a contour plot of the scaled imaginary part, meaning the height values of the left graphic translate into color values in the right graphic. Red is smallest and violet is largest. Along the real axis, the imaginary part of 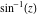 vanishes identically in the interval vanishes identically in the interval 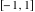 . Going away from the real axis into the . Going away from the real axis into the  is a function that approaches is a function that approaches 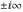 logarithmically. logarithmically. The absolute value of the imaginary part of 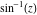 where where  . The surface is colored according to the absolute value of the real part. The right graphic is a contour plot of the scaled absolute value of the imaginary part, meaning the height values of the left graphic translate into color values in the right graphic. Red is smallest and violet is largest. Along the real axis, the imaginary part of . The surface is colored according to the absolute value of the real part. The right graphic is a contour plot of the scaled absolute value of the imaginary part, meaning the height values of the left graphic translate into color values in the right graphic. Red is smallest and violet is largest. Along the real axis, the imaginary part of 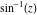 vanishes identically in the interval vanishes identically in the interval 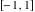 . Going away from the real axis into the . Going away from the real axis into the  is a function that approaches is a function that approaches 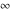 logarithmically. logarithmically. Absolute value part over the complex plane The absolute value of 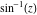 where where 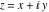 . The surface is colored according to the argument. The right graphic is a contour plot of the scaled absolute value, meaning the height values of the left graphic translate into color values in the right graphic. Red is smallest and violet is largest. Away from the origin, the absolute value of . The surface is colored according to the argument. The right graphic is a contour plot of the scaled absolute value, meaning the height values of the left graphic translate into color values in the right graphic. Red is smallest and violet is largest. Away from the origin, the absolute value of 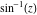 is logarithmically increasing. is logarithmically increasing. Argument over the complex plane The argument of 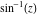 where where 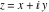 . The surface is colored according to the absolute value. The right graphic is a contour plot of the scaled argument, meaning the height values of the left graphic translate into color values in the right graphic. Red is smallest and violet is largest. . The surface is colored according to the absolute value. The right graphic is a contour plot of the scaled argument, meaning the height values of the left graphic translate into color values in the right graphic. Red is smallest and violet is largest.  has lines of discontinuities over the has lines of discontinuities over the 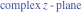 . . The square of the sine of the argument of 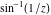 where where  . For dominantly real values, the function values are near 0, and for dominantly imaginary values, the function values are near 1. The surface is colored according to the absolute value. The right graphic is a cyclically colored contour plot of the argument. Red represents arguments near . For dominantly real values, the function values are near 0, and for dominantly imaginary values, the function values are near 1. The surface is colored according to the absolute value. The right graphic is a cyclically colored contour plot of the argument. Red represents arguments near 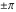 and light‐blue represents arguments near 0. and light‐blue represents arguments near 0. Zero-pole plot The logarithm of the absolute value of 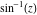 where where 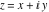 in the upper half‐plane. The surface is colored according to the square of the argument. In this plot zeros are easily visible as spikes extending downwards and poles as spikes extending upwards. The zero at in the upper half‐plane. The surface is colored according to the square of the argument. In this plot zeros are easily visible as spikes extending downwards and poles as spikes extending upwards. The zero at 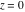 is clearly visible. is clearly visible. Real part over the complex plane near infinity The real part of 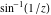 where where 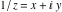 . The surface is colored according to the imaginary part. The right graphic is a contour plot of the scaled real part, meaning the height values of the left graphic translate into color values in the right graphic. Red is smallest and violet is largest. At . The surface is colored according to the imaginary part. The right graphic is a contour plot of the scaled real part, meaning the height values of the left graphic translate into color values in the right graphic. Red is smallest and violet is largest. At 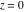 the function the function 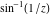 has a logarithmic singularity. has a logarithmic singularity. The absolute value of the real part of 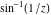 where where 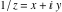 . The surface is colored according to the absolute value of the imaginary part. The right graphic is a contour plot of the scaled absolute value of the real part, meaning the height values of the left graphic translate into color values in the right graphic. Red is smallest and violet is largest. At . The surface is colored according to the absolute value of the imaginary part. The right graphic is a contour plot of the scaled absolute value of the real part, meaning the height values of the left graphic translate into color values in the right graphic. Red is smallest and violet is largest. At 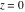 the function the function 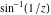 has a logarithmic singularity. has a logarithmic singularity. Imaginary part over the complex plane near infinity The imaginary part of 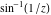 where where  . The surface is colored according to the real part. The right graphic is a contour plot of the scaled imaginary part, meaning the height values of the left graphic translate into color values in the right graphic. Red is smallest and violet is largest. At . The surface is colored according to the real part. The right graphic is a contour plot of the scaled imaginary part, meaning the height values of the left graphic translate into color values in the right graphic. Red is smallest and violet is largest. At 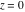 the function the function 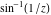 has a logarithmic singularity. has a logarithmic singularity. The absolute value of the imaginary part of 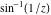 where where 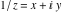 . The surface is colored according to the absolute value of the real part. The right graphic is a contour plot of the scaled absolute value of the imaginary part, meaning the height values of the left graphic translate into color values in the right graphic. Red is smallest and violet is largest. At . The surface is colored according to the absolute value of the real part. The right graphic is a contour plot of the scaled absolute value of the imaginary part, meaning the height values of the left graphic translate into color values in the right graphic. Red is smallest and violet is largest. At 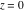 the function the function 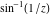 has a logarithmic singularity. has a logarithmic singularity. Absolute value part over the complex plane near infinity The absolute value of  where where 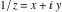 . The surface is colored according to the argument. The right graphic is a contour plot of the scaled absolute value, meaning the height values of the left graphic translate into color values in the right graphic. Red is smallest and violet is largest. At . The surface is colored according to the argument. The right graphic is a contour plot of the scaled absolute value, meaning the height values of the left graphic translate into color values in the right graphic. Red is smallest and violet is largest. At 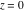 the function the function 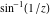 has a logarithmic singularity. has a logarithmic singularity. Argument over the complex plane near infinity The argument of 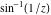 where where 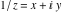 . The surface is colored according to the absolute value. The right graphic is a contour plot of the scaled argument, meaning the height values of the left graphic translate into color values in the right graphic. Red is smallest and violet is largest. At . The surface is colored according to the absolute value. The right graphic is a contour plot of the scaled argument, meaning the height values of the left graphic translate into color values in the right graphic. Red is smallest and violet is largest. At 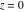 the function the function 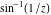 has a logarithmic singularity. has a logarithmic singularity. The square of the sine of the argument of 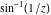 where where 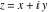 . For dominantly real values, the function values are near 0, and for dominantly imaginary values, the function values are near 1. The surface is colored according to the absolute value. The right graphic is a cyclically colored contour plot of the argument. Red represents arguments near . For dominantly real values, the function values are near 0, and for dominantly imaginary values, the function values are near 1. The surface is colored according to the absolute value. The right graphic is a cyclically colored contour plot of the argument. Red represents arguments near 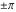 and light‐blue represents arguments near 0. and light‐blue represents arguments near 0. Zero-pole plot near infinity The logarithm of the absolute value of 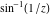 where where 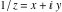 in the upper half plane. The surface is colored according to the square of the argument. In this plot zeros are easily visible as spikes extending downwards and poles and logarithmic singularities as spikes extending upwards. At in the upper half plane. The surface is colored according to the square of the argument. In this plot zeros are easily visible as spikes extending downwards and poles and logarithmic singularities as spikes extending upwards. At 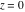 the function the function 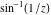 has a logarithmic singularity. has a logarithmic singularity.
|