|
The elliptic exponent 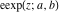 , its derivative , its derivative 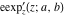 , and the elliptic logarithm , and the elliptic logarithm 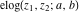 have the following values at the origin point: have the following values at the origin point:
The elliptic exponent 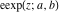 has the following value at the specialized point has the following value at the specialized point 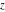 : :
The elliptic exponent 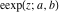 and its derivative and its derivative 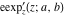 are vector‐valued functions of are vector‐valued functions of 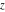 , , 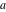 , and , and 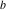 , which are analytic in each component, and they are defined over , which are analytic in each component, and they are defined over 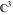 . .
The elliptic logarithm 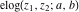 is an analytical function of is an analytical function of 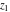 , , 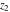 , , 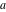 , ,  , which is defined in , which is defined in 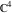 . .
The elliptic exponent 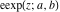 , its derivative , its derivative 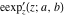 , and the elliptic logarithm , and the elliptic logarithm 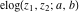 have complicated branch cuts. have complicated branch cuts.
The elliptic logarithm 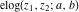 does not have poles and essential singularities. does not have poles and essential singularities.
The elliptic exponent  , its derivative , its derivative 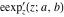 , and the elliptic logarithm , and the elliptic logarithm 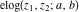 do not have periodicity. do not have periodicity.
The elliptic exponent 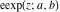 , its derivative , its derivative 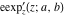 , and the elliptic logarithm , and the elliptic logarithm 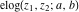 have mirror symmetry: have mirror symmetry:
The elliptic logarithm 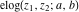 has the following integral representation: has the following integral representation:
The elliptic exponent 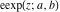 satisfies the following identities including the complete elliptic integral satisfies the following identities including the complete elliptic integral  : :
The first derivatives of elliptic exponent 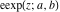 and the elliptic logarithm and the elliptic logarithm 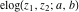 have the following representations: have the following representations:
The elliptic exponent 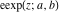 , its derivative , its derivative 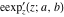 , and the elliptic logarithm , and the elliptic logarithm 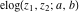 satisfy the following ordinary nonlinear differential equations: satisfy the following ordinary nonlinear differential equations:
|