|
Introduction to the Gamma Function
General
The gamma function 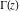 is used in the mathematical and applied sciences almost as often as the well-known factorial symbol is used in the mathematical and applied sciences almost as often as the well-known factorial symbol 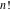 . It was introduced by the famous mathematician L. Euler (1729) as a natural extension of the factorial operation . It was introduced by the famous mathematician L. Euler (1729) as a natural extension of the factorial operation 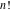 from positive integers from positive integers 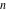 to real and even complex values of the argument to real and even complex values of the argument 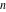 . This relation is described by the following formula: . This relation is described by the following formula:
L. Euler derived some basic properties and formulas for the gamma function. He started investigations of 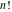 from the infinite product from the infinite product
and the integral
which is currently known as the beta function integral. As a result, Euler derived the following integral representation for factorial 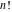 : :
which can be easily converted into the well-known Euler integral for the gamma function:
Also, during his research, Euler closely approached the famous reflection formula:
which later got his name.
At the same time, J. Stirling (1730) found the famous asymptotic formula for the factorial, which bears his name. This formula was also naturally applied to the gamma function resulting in the following asymptotic relation:
Later, A. M. Legendre (1808, 1814) suggested the current symbol Γ for the gamma function and discovered the duplication formula:
It was generalized by C. F. Gauss (1812) to the multiplication formula:
F. W. Newman (1848) studied the reciprocal of the gamma function and found that it is an entire function and has the following product representation valid for the whole complex plane:
where 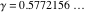 is the Euler–Mascheroni gamma constant. is the Euler–Mascheroni gamma constant.
B. Riemann (1856) proved an important relation between the gamma and zeta functions:
which was mentioned centuries ago in an article by Euler (1749) for particular values of the argument 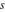 . .
K. Weierstrass (1856) and other nineteenth century mathematicians widely used the gamma function in their investigations and discovered many more complicated properties and formulas for it. In particular, H. Hankel (1864, 1880) derived its contour integral representation for complex arguments, and O. Hölder (1887) proved that the gamma function does not satisfy any algebraic differential equation. This result was subsequently re-proved by A. Ostrowski (1925).
Many mathematicians devote special attention to the question of the uniqueness of extending the factorial operation 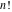 from positive integers to arbitrary real or complex values. Evidently this question is connected to the solutions of the functional equation: from positive integers to arbitrary real or complex values. Evidently this question is connected to the solutions of the functional equation:
J. Hadamard (1894) found that the function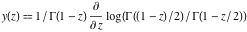 is an entire analytic function that coincides with is an entire analytic function that coincides with 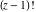 for for  . But this function satisfies the more complicated functional equation . But this function satisfies the more complicated functional equation 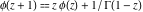 and has a more complicated integral representation than the classical gamma function defined by the Euler integral. and has a more complicated integral representation than the classical gamma function defined by the Euler integral.
H. Bohr and J. Mollerup (1922) proved that the gamma function 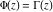 is the only function that satisfies the recurrence relationship is the only function that satisfies the recurrence relationship 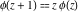 , is positive for , is positive for 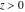 , equals one at , equals one at 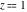 , and is logarithmically convex (that is, , and is logarithmically convex (that is, 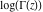 is convex). If the restriction on convexity is absent, then the recurrence relationship has an infinite set of solutions in the form is convex). If the restriction on convexity is absent, then the recurrence relationship has an infinite set of solutions in the form 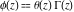 , where , where 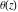 is an arbitrary periodic function with period is an arbitrary periodic function with period 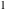 . .
Definition of gamma function
The gamma function 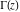 in the half-plane in the half-plane 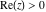 is defined as the value of the following definite integral: is defined as the value of the following definite integral:
This integral is an analytic function that can be represented in different forms; for example, as the following sum of an integral and a series without any restrictions on the argument:
The last formula can also be used as an equivalent definition of the gamma function.
A quick look at the gamma function Here is a quick look at the graphics for the gamma function along the real axis.
Connections within the group of gamma functions and with other function groups
Representations through more general functions
The gamma function 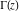 is the main example of a group of functions collectively referred to as gamma functions. For example, it can be written in terms of the incomplete gamma function: is the main example of a group of functions collectively referred to as gamma functions. For example, it can be written in terms of the incomplete gamma function:
All four incomplete gamma functions  , , 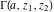 , , 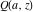 , and , and 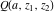 can be represented as cases of the hypergeometric function can be represented as cases of the hypergeometric function 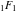 . Further, the gamma function Γ(z) is the special degenerate case of the hypergeometric function . Further, the gamma function Γ(z) is the special degenerate case of the hypergeometric function 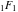 . .
Representations through related equivalent functions
The gamma function 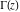 and two factorial functions are connected by the formulas: and two factorial functions are connected by the formulas:
The best-known properties and formulas for the gamma function
Values at points
The gamma function 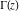 can be exactly evaluated in the points can be exactly evaluated in the points 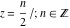 . Here are examples: . Here are examples:
Specific values for specialized variables
The preceding evaluations can be provided by the formulas:
At the points 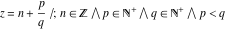 , the values of the gamma function , the values of the gamma function 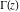 can be represented through values of can be represented through values of  : :
Real values for real arguments
For real values of argument  , the values of the gamma function , the values of the gamma function 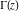 are real (or infinity). The gamma function is not equal to zero: are real (or infinity). The gamma function is not equal to zero:
Analyticity
The gamma function 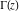 is an analytical function of is an analytical function of 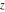 , which is defined over the whole complex , which is defined over the whole complex 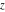 ‐plane with the exception of countably many points ‐plane with the exception of countably many points 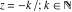 . The reciprocal of the gamma function . The reciprocal of the gamma function 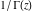 is an entire function. is an entire function.
Poles and essential singularities
The function 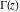 has an infinite set of singular points has an infinite set of singular points 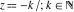 , which are the simple poles with residues , which are the simple poles with residues 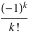 . The point . The point 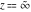 is the accumulation point of the poles, which means that is the accumulation point of the poles, which means that 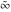 is an essential singular point. is an essential singular point.
Branch points and branch cuts
The function 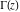 does not have branch points and branch cuts. does not have branch points and branch cuts.
Periodicity
The function 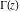 does not have periodicity. does not have periodicity.
Parity and symmetry
The function  has mirror symmetry: has mirror symmetry:
Differentiation
The derivatives of 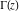 can be represented through gamma and polygamma functions: can be represented through gamma and polygamma functions:
Ordinary differential equation
The gamma function 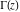 does not satisfy any algebraic differential equation (O. Hölder, 1887). But it is the solution of the following nonalgebraic equation: does not satisfy any algebraic differential equation (O. Hölder, 1887). But it is the solution of the following nonalgebraic equation:
Series representations
Series representations of the gamma function 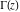 near the poles near the poles  are of great interest for applications in the theory of generalized hypergeometric, Meijer G, and Fox H functions. These representations can be described by the formulas: are of great interest for applications in the theory of generalized hypergeometric, Meijer G, and Fox H functions. These representations can be described by the formulas:
where 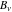 are the Bernoulli numbers. are the Bernoulli numbers.
Asymptotic series expansions
Asymptotic behavior of the gamma function 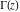 is described by the famous Stirling formula: is described by the famous Stirling formula:
This formula allows derivation of the following asymptotic expansion for the ratio of gamma functions:
Integral representations
The gamma function 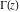 has several integral representations that are different from the Euler integral: has several integral representations that are different from the Euler integral:
and related integral
which can be used for defining the gamma function over the whole complex plane.
Some of the integral representations are the following:
This final formula is known as Hankel's contour integral. The path of integration 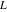 starts at starts at 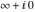 on the real axis, goes to on the real axis, goes to 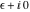 , circles the origin in the counterclockwise direction with radius , circles the origin in the counterclockwise direction with radius 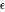 to the point to the point 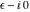 , and returns to the point , and returns to the point 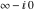 . .
Product representations
The following infinite product representation for 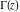 clearly illustrates that clearly illustrates that 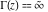 at at  : :
The similar product representation for 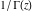 illustrates that illustrates that 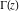 is an entire function: is an entire function:
Limit representations
The following famous limit representation for 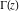 was known to L. Euler: was known to L. Euler:
It can be modified to the following related limit representations:
The gamma function can be evaluated as the limit of the following definite integral:
Definite integration
The most famous definite integrals, including the gamma function, belong to the class of Mellin–Barnes integrals. They are used to provide a uniform representation of all generalized hypergeometric, Meijer G, and Fox H functions. For example, the Meijer G function is defined as the value of the following Mellin–Barnes integral:
The infinite contour of integration 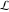 separates the poles of separates the poles of 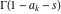 at at 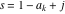 , , 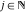 from the poles of from the poles of 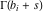 at at 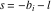 , , 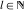 . Such a contour always exists in the cases . Such a contour always exists in the cases 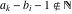 . .
There are three possibilities for the contour  : :
(i)  runs from γ-ⅈ∞ to γ+ⅈ∞ (where runs from γ-ⅈ∞ to γ+ⅈ∞ (where  ) so that all poles of ) so that all poles of 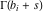 , , 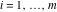 , are to the left of , are to the left of 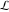 , and all the poles of , and all the poles of 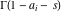 , , 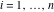 are to the right of ℒ. This contour can be a straight line are to the right of ℒ. This contour can be a straight line 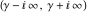 if if 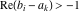 (then (then 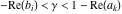 ). In this case, the integral converges if ). In this case, the integral converges if 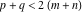 , , 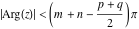 . If . If 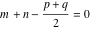 , then , then 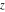 must be real and positive, and the additional condition must be real and positive, and the additional condition 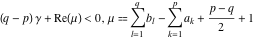 , should be added. , should be added.
(ii) 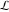 is a left loop, starting and ending at is a left loop, starting and ending at and encircling all poles of and encircling all poles of 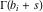 , , , once in the positive direction, but none of the poles of , once in the positive direction, but none of the poles of 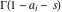 , , 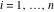 . In this case, the integral converges if . In this case, the integral converges if  and either and either 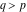 or or 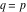 and and 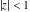 or or 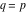 and and 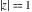 and and 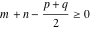 and and 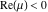 . .
(iii) 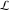 is a right loop, starting and ending at +∞ and encircling all poles of is a right loop, starting and ending at +∞ and encircling all poles of 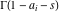 , , 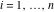 , once in the negative direction, but none of the poles of , once in the negative direction, but none of the poles of  , , 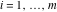 . In this case, the integral converges if . In this case, the integral converges if 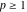 , and either , and either 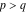 or or 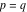 and and 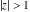 or or 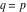 and and 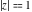 and and 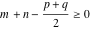 and and 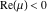 . .
In particular cases, the last integral can be evaluated using simpler elementary and special functions:
Integral transforms
The definition of the Meijer G function through a Mellin‐Barnes integral realizes the inverse Mellin integral transform of ratios of gamma functions:
The contour  is the vertical straight line is the vertical straight line 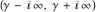 . It allows the writing of the following rather general formula for the inverse Mellin integral transform: . It allows the writing of the following rather general formula for the inverse Mellin integral transform:
In particular cases, it gives the following representations:
Transformations
The following formulas describe some transformations of the gamma functions with linear arguments into expressions that contain the gamma function with the simplest argument:
Multiple arguments
In the case of multiple arguments 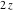 , , 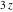 ,…, ,…, 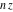 , the gamma function , the gamma function 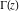 can be represented by the following duplication and multiplication formulas, derived by A. M. Legendre and C. F. Gauss: can be represented by the following duplication and multiplication formulas, derived by A. M. Legendre and C. F. Gauss:
Products involving the direct function
The product of two gamma functions 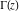 and and 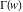 , with arguments satisfying the condition that , with arguments satisfying the condition that 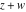 is an integer, can be represented through elementary functions: is an integer, can be represented through elementary functions:
The preceding formula transforms into the following formula and its relatives:
The ratio of two gamma functions 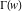 and and 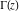 , with arguments satisfying the condition that , with arguments satisfying the condition that 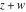 is integer, can be represented through a polynomial or rational function: is integer, can be represented through a polynomial or rational function:
Identities
The gamma function 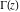 satisfies the following recurrence identities: satisfies the following recurrence identities:
These formulas can be generalized to the following recurrence identities with a jump of length  : :
Inequalities
The most famous inequalities for the gamma function can be described by the following formulas:
Applications
The gamma function is used throughout mathematics, the exact sciences, and engineering.
|